Temporal coherent control of resonant two-photon double ionization of the hydrogen molecule via doubly excited states

Authors

Jean Marcel Ngoko Djiokap, University of Nebraska-LincolnFollow
Anthony F. Starace, University of Nebraska-LincolnFollow

ORCID IDs

J. M. Ngoko Djiokap https://orcid.org/0000-0003-4702-3544

Document Type

Article

Date of this Version

2021

Citation

PHYSICAL REVIEW A 103, 053110 (2021)

DOI: 10.1103/PhysRevA.103.053110

Comments

©2021 American Physical Society

Abstract

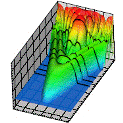
We use time-delayed, counter-rotating, circularly polarized few-cycle attosecond nonoverlapping pulses to study the temporal coherent control of the resonant process of two-photon double ionization (TPDI) of hydrogen molecule via doubly excited states for pulse propagation direction along ˆk either parallel or perpendicular to the molecular axis ˆR. For ˆk ‖ ˆR and a pulse carrier frequency of 36 eV resonantly populating the Q₂ 1∏ ⁺ _u (1) doubly excited state as well as other 1∏ ⁺ _u doubly excited states, we find that the indirect ionization pathway through these doubly excited states changes the character of the kinematical vortex-shaped momentum distribution produced by the two direct ionization pathways from fourfold to twofold rotational symmetry. This result is similar to what found in TPDI of the He atom involving 1Po± 1 doubly excited states [Ngoko Djiokap and Starace, J. Opt. 19, 124003 (2017)]; however, angular distributions exhibiting a quantum beat effect between the ground state and a doubly excited state seen for the He atom are observed here for its molecular counterpart with an anomaly in shape and magnitude, not in frequency. The sixfold differential probability integrated over the azimuthal angle of the photoelectron pair shows that this anomaly is due to autoionization decays and quantum beats between doubly excited states. For ˆk ⊥ ˆR and a broadband pulse carrier frequency of 30 eV populating the Q₁ 1∏ ⁺ _u (1), Q₁ 1∑ ⁺ _u (1), Q₂ 1∏ ⁺ _u (1), and Q₁ 1∑ ⁺ _u (2) doubly excited states, the momentum distribution is shown to exhibit dynamical electron vortices with four spiral arms, which originates from the interplay between the ¹Δ ⁺ _g , ¹Π⁺ _g , and ¹Σ ⁺ _g dynamical ionization amplitudes. Our treatment within either the adiabatic-nuclei approximation or fixed-nuclei approximation shows that the latter provides a very good account for this correlated process.